
Almost every large business borrows money. The team leader for borrowings is normally the treasurer. The treasurer must safeguard the firm’s cash flows at all times, as well as understand and manage the impact of borrowings on the company’s interest costs and profits. So treasurers need a deep and joined-up understanding of the effects of different borrowing structures, both on the firm’s cash flows and on its profits. Negotiating the circularity of equal loan instalments can feel like being lost in a maze. Let's take a look at practical cash and profit management.
CASH IS KING
Say we borrow £10m in a lump sum, to be repaid in annual instalments. Obviously, the lender requires full repayment of the £10m principal (capital) borrowed. They will also require interest.
Let’s say the rate of interest is 5% per year.
The first year’s interest, before any repayments, is simply the original £10m x 5% = £0.5m
The expense charged to the income statement, reducing net profits for the first year, is £0.5m.
But the next year can start to seem complicated.
BUSINESS DILEMMA
Our instalment will repay some of the principal, as well as paying the interest. This means the second year’s interest charge will be less than the first, because of the principal repayment. But what if we can’t afford larger instalments in the earlier years? Can we make our total cash outflows the same in each year? Is there an equal instalment that will repay just the right amount of principal in each year, to leave the original borrowing repaid, together with all of the reducing annual interest charges, by the end?
CIRCLE SOLVER
Help is at hand. There is, indeed, an equal instalment that does just that, sometimes called an equated instalment. Equated instalments pay off varying proportions of interest and principal within each period, so that by the end, the loan has been paid off in full.
The equated instalments deal nicely with our cash flow problem, but the interest charges still seem complicated.
|
Equated instalment
|
DYNAMIC BALANCE
As we’ve seen, interest is only charged on the reducing balance of the principal. So the interest charge per period starts out relatively large, and then it gets smaller with each annual repayment.
The interest calculation is potentially complicated, even circular, because our principal repayments are changing as well. As the interest element of the instalment goes down each year, the balance available to pay off the principal is going up every time.
How can we figure out the varying annual interest charges? Let’s look at this example:
Southee Limited, a construction company, is planning to acquire new earth-moving equipment at a cost of £10m. Southee is considering a bank loan for the full cost of the equipment, repayable over four years in equal annual instalments, incorporating interest at a rate of 5% per annum, the first instalment to be paid one year from the date of taking out the loan.
You need to be able to calculate the annual instalment that would be payable under the bank loan, calculate how much would represent the principal repayment and also how much would represent interest charges, in each of the four years and in total.
In other words, you need to be able to work out these five things:
(1) The annual instalment
(2) Total principal repayments
(3) Total interest charges
(4) Interest charges for each year
(5) Principal repayments in each year
ANNUAL INSTALMENT
The best place to start is with the annual instalment. To work out the annual instalment we need an annuity factor. The annuity factor (AF) is the ratio of our equated annual instalment, to the principal of £10m borrowed at the start.
The annuity factor itself is calculated as:
AF = (1 – (1+r)-n ) ÷ r
Where:
r = interest rate per period = 0.05 (5%)
n = number of periods = 4 (years)
Applying the formula:
AF = (1 – 1.05-4 ) ÷ 0.05 = 3.55
Now, the equated annual instalment is given by:
Instalment = Principal ÷ annuity factor = £10m ÷ 3.55 = £2.82m
TOTAL PRINCIPAL REPAYMENTS
The total of the principal repayments is simply the total principal originally borrowed, ie £10m.
TOTAL INTEREST CHARGES
The total of the interest charges is the total of all the repayments, minus the total principal repaid. We’re only paying principal and interest, so any amount paid that isn’t principal, must be interest.
There are four payments of £2.82m each.
So the total repayments are: £2.82m x 4 = £11.3m
And the total interest charges for the four years are: £11.3m less £10m = £1.3m
Now we need to allocate this £1.3m total across each of the four years.
INTEREST CHARGES FOR EACH YEAR
The allocations are easier to figure out in a nice table. Let’s invest a little time in one, filling in the figures we already know. (All amounts are in £m.)
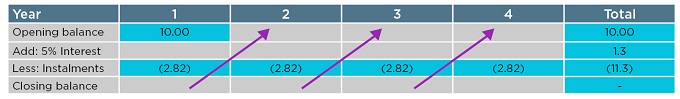
The closing balance for each year will be the opening balance for the next year.
By the time we get to the end of the fourth year, we’ll have repaid the whole of the £10m originally borrowed, together with a total of £1.3m interest.
PRINCIPAL REPAYMENTS IN EACH YEAR
We can now fill in the 5% interest per year, and all our figures will flow through nicely.
We’ve already calculated the interest charge for the first year:
0.05 x £10m = £0.5m
So our closing balance for the first year is:
Opening balance + interest – instalment = 10.00 + 0.5 – 2.82 = £7.68m
So we can go on to fill in the rest of our table, as set out below:

(There is a minor rounding difference of £0.01m in year four that we don’t need to worry about. It would disappear if we used more decimal places.)
____________________
Author: Doug Williamson
Source: The Treasurer magazine
Want to learn more about treasury and cash management?
Choose from:
• eLearning courses: 45-90 minutes to complete, available online 24/7.
• Training courses: live skills-based training sessions.
• Treasury and Cash Management qualifications: internationally recognised courses from entry to master level.
All our courses will provide you with valuable knowledge as well as easy-to-share digital credentials to demonstrate your learning achievements.
